
If the order between complex numbers is complete (i.e. But, as we just saw, the order between complex numbers for which (1) holds, could not satisfy the law of trichotomy and vice versa. Trichotomy holds for the usual order of real numbers. The law by which for any two numbers a and b, either a < b or b < a or a = b, is known as Trichotomy.
The real problem stems from the sheer fact that i is somehow comparable to 0. Had we had i < 0 we would have gotten a contradiction in precisely the same way now applying 0 < (-i). The crucial thing was that i satisfied the inequality 0 < i. The derivation is quite formal and does not use any particular properties of the lexicographic order. By definition, this implies 1 < (-1) which is absurd. Multiplying by i one more time produces -i 2 < i 2. Assume (1) holds for complex numbers and the lexicographic order. Note that i may be written as 0 + i1 while 0 = 0 + i0. The following is true for real numbers: (1) To see what the problem is let's turn to the multiplication.

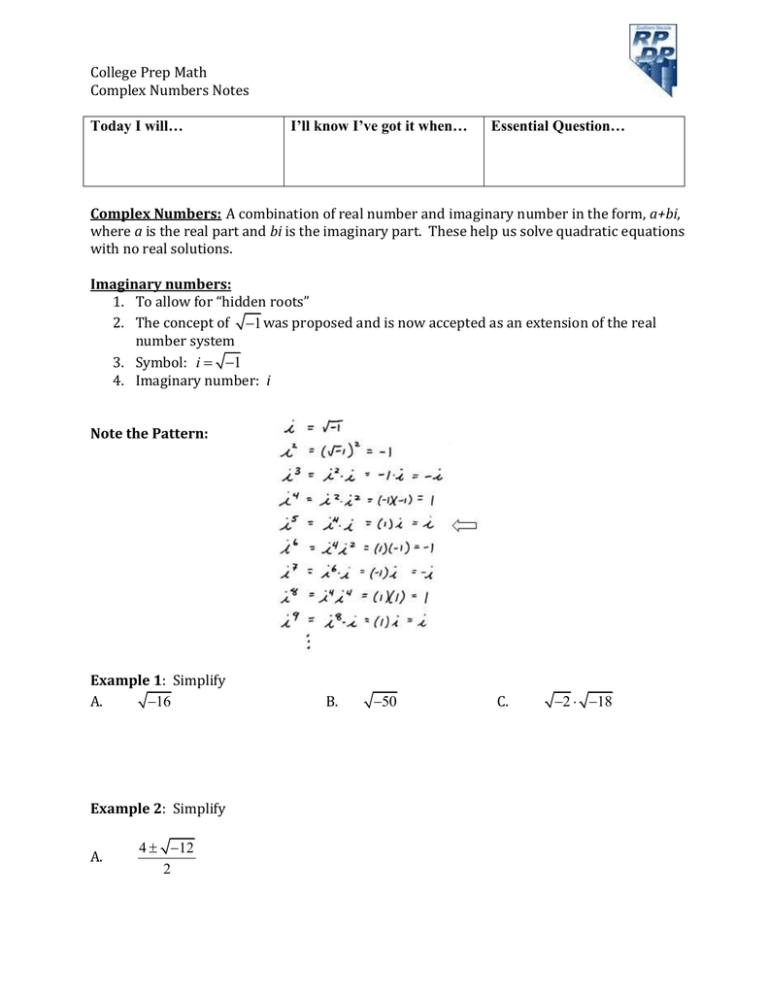
(1 + 4i) < (5 - 2i) which is of course true. The same is true for complex numbers as well. For example, if r < s and t < u then (r + t) < (s + u), where r, s, t, u are real numbers. Help With Complex Numbers 2 Thread starter jisbon Start date 1 2 Next 1 of 2 Go to page Go Next Last 1 jisbon 467 30 Homework Statement: NIL Relevant Equations: NIL -Continued-Spoiler: Solved. Additionally, for real number, the relation "<" is related to the arithmetic operations "+" and "×". This relation, exactly like in the case of integers or real numbers, exhibits the following properties:Īlso, since a real number x can be identified with the complex number x + i0, we immediately see that thus defined order conforms to the usual order of real numbers. This is how words are ordered in dictionaries. This is what is called the lexicographic order. Provided either a < c or a = c and b < d. It's actually possible to define the relationship We must be cautious with this kind of general statements. But you can't compare two complex numbers. Last, we need to divide the entire numerator by 13.Among any two integers or real numbers one is larger, another smaller. Let’s now place these expressions in a fraction where they belong. To multiply, two tables need to be set up – one for multiplying the numerators and the other for multiplying the denominators, like so. When multiplying fractions, multiply left-to-right. We multiplied the numerator by the same amount so as not to alter the value of the original fraction. We will do so by multiplying the denominator by its complex conjugate, 3 – 2i, as follows. The only way to clean up this division problem is to cancel the imaginary numbers in the denominator. To divide these complex umbers, we are going to employ a slight trick. The same problem can be written vertically, like a fraction. Here is an example of a division problem.
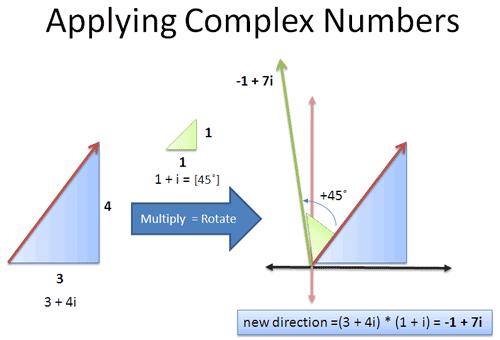
We will make use of this interesting phenomenon when we divide complex numbers. Here is a table already set up and filled.Įvery time we multiply complex conjugates, the imaginary term cancels and we obtain a real answer. Here is an example of two complex conjugates and we are going to multiply them. When conjugates are multiplied together, something interesting happens. Here is a table that has complex numbers and their corresponding conjugates. To deal with it, we have to deal with something called conjugates.Ī conjugate of a complex number is gained by taking the opposite of the imaginary part of a complex number. Watch our video and try our quizmaster on this lesson.ĭividing complex numbers is a bit technical. Here are the terms from the table and the subsequent steps for simplifying the expression. Here is the set-up for using a multiplication table. We can clean up the expression as follows. We now need to make a substitution for i-squared. Next, let’s arrange all the terms together from our table. Now, we need to start multiplying columns times rows. We will place the first complex number along the top of the table and the left side of the table. Doing so helps us to organize our information. To multiply these complex numbers, we will use a multiplication table. It is completely possible that a a or b b could be zero and so in 16 i i the real part is zero. The last two probably need a little more explanation. Multiplying complex numbers requires more detail than adding or subtracting complex numbers. Here are some examples of complex numbers.


 0 kommentar(er)
0 kommentar(er)
